Chapter 8 Quadrilaterals Exercise 8.2
Question 1: ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA (see Fig 8.29). AC is a diagonal. Show that:i) SR || AC and SR = 1/2 AC
ii) PQ = SR
iii) PQRS is a parallelogram

i) In ∆ADC, S and R are the midpoints of AD and CD respectively.
Therefore, by midpoint theorem (the line segment joining the midpoints of two sides of a triangle is parallel to the third side and also half of it).
∴ SR || AC and SR = 1/2 AC …(1)
ii) In ∆ABC,
P and Q are midpoints of AB and BC respectively.
Therefore, by midpoint theorem,
∴ PQ || AC and PQ = 1/2 AC …(2)
From (1) and (2),
PQ || SR and PQ = SR …(3)
iii) From (3), PQ || SR and PQ = SR. As one pair of opposite sides of quadrilateral PQRS is equal and parallel.
Hence PQRS is a parallelogram.
Question 2: ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
Answer:

In ∆ABC, P and Q are the midpoint of AB and BC respectively.
→ PQ || AC and PQ = 1/2 AC (by midpoint theorem) …(1)
In ∆ADC, R and S are the midpoint of CD and AD respectively.
→ RS || AC and RS = 1/2 AC (by midpoint theorem) …(2)
From (1) and (2),
PQ || RS and PQ = RS
Since in quadrilateral PQRS, one pair of opposite sides is equal and parallel to each other, it is a parallelogram.
Let the diagonals of rhombus ABCD intersect each other at O.
In quadrilateral OMQN,
MQ || ON (∵ PQ || AC)
QN || OM (∵ QR || BD)
∴ OMQN is a parallelogram
→ ∠MQN = ∠NOM
→ ∠PQR = ∠NOM
However, ∠NOM = 90° (diagonals of a rhombus are perpendicular to each other)
Hence ∠PQR = 90°
PQRS is a parallelogram with one of its interior angles as 90°. Hence, PQRS is a rectangle.
Question 3: ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
Answer:

Let us join BD and AC.
In ∆ABC, P and Q are midpoints of AB and BC respectively.
→ PQ || AC and PQ = 1/2 AC (by mid point theorem) …(1)
Similarly, in ∆ADC, S and R are midpoints of AD and DC respectively.
→ SR || AC and SR = 1/2 AC (by mid point theorem) …(2)
From (1) and (2), PQ || SR and PQ = SR
Since in quadrilateral PQRS, one pair of opposite sides is equal and parallel to each other, it is a parallelogram.
→ PS || QR and PS = QR (opposite sides of parallelogram) …(3)
In ∆BCD, Q and R are midpoints of BC and CD respectively.
→ QR || BD and QR = 1/2 BD (by midpoint theorem) …(4)
However, the diagonals of a rectangle are equal.
→ AC = BD …(5)
From (1), (2), (3), (4) and (5), we get
PQRS is a rhombus.
Question 4: ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F (see Fig. 8.30). Show that F is the mid-point of BC.

In ∆ABD, EF || AB and E is the midpoint of AD.
∴ G will be midpoint of DB (as EF || AB and AB || CD).
Hence, EF || CD (two lines are parallel to each other)
In ∆BCD, GF || CD and G is midpoint of BD.
∴ By converse of midpoint theorem, F is midpoint of BC.
Question 5: In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively (see Fig. 8.31). Show that the line segments AF and EC trisect the diagonal BD.
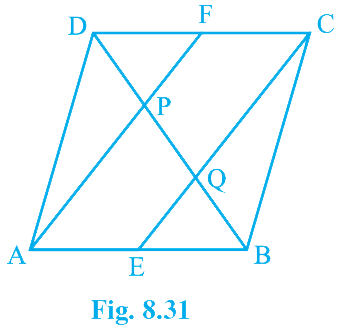
→ AB || CD
Hence, AE || FC
Again AB = CD (opposite sides of parallelogram ABCD)
= 1/2 AB = 1/2 CD
= AE = FC (as E & F are midpoints of AB and CD respectively)
In quadrilateral AECF, one pair of opposite sides (AE and FC) are parallel and equal to each other.
∴ AECF is a parallelogram.
= AF || EC (opposite sides of parallelogram AECF)
In ∆DQC, F is midpoint of DC and FP || CQ (as AF || EC)
∴ By converse of midpoint theorem, it can be said that P is the midpoint of DQ
Therefore, DP = PQ …(1)
In ∆APB, E is the midpoint of AB and EQ || AP (as AF || EC)
Therefore by converse of midpoint theorem, it can be said that Q is the midpoint of PB.
Therefore, PQ = QB …(2)
From (1) and (2),
DP = PQ = BQ
Hence the line segment AF and EC trisect the diagonal BD.
Question 6: Show that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other.
Answer:

In ∆ABD, S and P are midpoints of AD and AB respectively.
Therefore, by midpoint theorem,
SP || BD and SP = 1/2 BD …(1)
In ∆BCD, Q and R are midpoints of BC and CD respectively.
Therefore, by midpoint theorem,
QR || BD and QR = 1/2 BD …(2)
From (1) and (2)
SP || QR and SP = QR
In quadrilateral SPQR, one pair of opposite sides is equal and parallel to each other.
∴ SPQR is a parallelogram.
We know that the diagonals of a parallelogram bisect each other.
Therefore, PR and QS bisect each other.
Question 7: ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that
i) D is the mid-point of AC
ii) MD ⊥ AC
(iii) CM = MA = 1/2 AB
Answer:

Therefore, D is the midpoint of AC (by converse of midpoint theorem)
ii) As DM || CB and AC is a transversal
→ ∠MDC + ∠DCB = 180° (co-interior angles)
= ∠MDC + 90° = 180°
= ∠MDC = 90°
→ MD ⊥ AC
iii) Join CM.
In ∆AMD and ∆CMD,
AD = AD (D is midpoint of AC)
∠ADM = ∠CDM (each 90°)
DM = DM (common)
Therefore, by SAS congruency, ∆AMD ≅ ∆CMD.
By CPCT, AM = CM
However, AM = 1/2 AB (as M is the midpoint of AB)
Therefore, it can be said that
CM = AM = 1/2 AB
No comments:
Post a Comment