NCERT Class 9 Maths Chapter 13 Surface Areas and Volumes Exercise 13.7

Chapter 13 Surface Areas and Volumes Exercise 13.7

Question 1: Find the volume of the right circular cone with
i) radius 6 cm, height 7 cm
ii) radius 3.5 cm, height 12 cm
Answer:
i) Given
Radius of the cone = 6 cm
Height of the cone = 7 cm
Volume of the cone = ?
Volume of the cone = 1/3πr²h
= 1/3 × 22/7 × 6 × 6 × 7
= 22 × 2 × 6
= 264 cm³

ii) Given
Radius of the cone = 3.5 cm
Height of the cone = 12 cm
Volume of the cone = ?
Volume of the cone = 1/3πr²h
= 1/3 × 22/7 × 3.5 × 3.5 × 12
= 22 × 0.5 × 3.5 × 4
= 154 cm³

Question 2: Find the capacity in litres of a conical vessel with
i) radius 7 cm, slant height 25 cm
ii) height 12 cm, slant height 13 cm
Answer:
i) Given
Radius of the cone = 7 cm
Slant height of the cone = 25 cm
Height of the cone = √25² - 7² = √625 - 49 = √576 = 24 cm
Volume of cone = 1/3πr²h
= 1/3 × 22/7 × 7 × 7 × 24
= 22 × 7 × 8
= 1232 cm³
1 l = 1000 cm³
So, 1232 cm³ = 1232/1000 = 1.232 l

ii) Given
Height of the cone = 12 cm
Slant height of the cone = 13 cm
Radius of the cone = √13² - 12² = √169 - 144 = √25 = 5 cm
Volume of cone = 1/3πr²h
= 1/3 × 22/7 × 5 × 5 × 12
= 22/7 × 5 × 5 × 4
= 2200/7 cm³
= 314.28 cm³
1 l = 1000 cm³
So, 314.28 cm³ = 314.28/1000 = 0.31428 l

Question 3: The height of a cone is 15 cm. If its volume is 1570 cm³, find the radius of the base. (Use π = 3.14)
Answer:
Given
Height of the cone = 15 cm
Volume of the cone = 1570 cm³
Radius of the base of the cone =?
Volume of cone = 1/3πr²h
1570 = 1/3 × 3.14 × r² × 15
1570 = 15.7r²
r² = 1570/15.7
r² = 100
r = √100
r = 10 cm
Therefore, the radius of the base of the cone is 10 cm.

Question 4: If the volume of a right circular cone of height 9 cm is 48π cm³, find the diameter of its base.
Answer:
Given
Volume of cone = 48π cm³
Height of the cone = 9 cm
Diameter of the base = ?
Volume of the cone = 1/3πr²h
48π = 1/3× π × r² × 9
48π = 3πr²
r² = 48π/3π
r² = 16
r = √16
r = 4 cm
Diameter = 2 × Radius = 2 × 4 = 8 cm
Therefore, the diameter of the base of the cone is 8 cm.

Question 5: A conical pit of top diameter 3.5 m is 12 m deep. What is its capacity in kilolitres?
Answer:
Given
Diameter of the conical pit = 3.5 m
Height of the conical pit = 12 m
Capacity in kilolitres = ?
Radius = Diameter/2 = 3.5/2 m
Volume of cone = 1/3πr²h
= 1/3 × 22/7 × 3.5/2 × 3.5/2 × 12
= 11 × 0.5 × 3.5 × 2
= 38.5 m³
1 kilolitre = 1 m³
38.5 m³ = 38.5 kl
Therefore, the capacity of the conical pit in kilolitres is 38.5 kl.

Question 6: The volume of a right circular cone is 9856 cm³. If the diameter of the base is 28 cm, find
i) height of the cone
ii) slant height of the cone
iii) curved surface area of the cone
Answer:
Given
Volume of the cone = 9856 cm³
Diameter of the base of the cone = 28 cm
Height of the cone = ?
Slant height of the cone = ?
Curved Surface Area of the cone = ?
Radius = Diameter/2 = 28/2 = 14 cm
Volume of the cone = 1/3πr²h
9856 = 1/3 × 22/7 × 14 × 14 × h
9856 = 1/3 × 22 × 2 × 14 × h
9856 = 616h/3
h = 9856 × 3/616
h = 48 cm
Slant height of cone = √r² + h²
= √(14)² + (48)²
= √196 + 2304
= √2500
= 50 cm
Curved Surface Area of cone = πrl
= 22/7 × 14 × 50
= 2200 cm²
Therefore, the height of the cone is 48 cm, slant height of the cone is 50 cm and the CSA of the cone is 2200 cm².

Question 7: A right triangle ABC with sides 5 cm, 12 cm and 13 cm is revolved about the side 12 cm. Find the volume of the solid so obtained.
Answer:

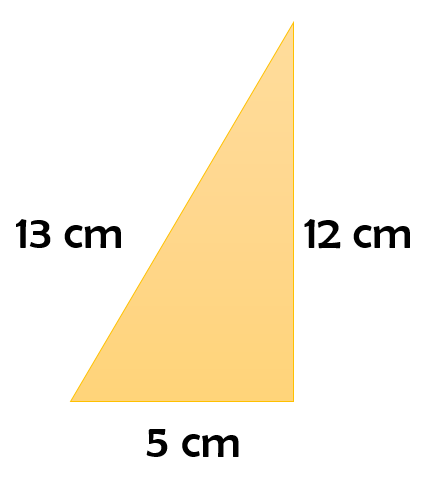
If a right angled triangle is revolved, then a cone is obtained.
Given
Radius of the cone = 5 cm
Height of the cone = 12 cm
Volume of the cone = 1/3πr²h
= 1/3 × π × 5 × 5 × 12
= 5 × 5 × 4 × π
= 100π cm³

Question 8: If the triangle ABC in the Question 7 above is revolved about the side 5 cm, then find the volume of the solid so obtained. Find also the ratio of the volumes of the two solids obtained in Questions 7 and 8.
Answer:

Given
Radius of the cone = 12 cm
Height of the cone = 5 cm
Volume of the cone = 1/3πr²h
= 1/3 × π × 12 × 12 × 5
= 5 × 12 × 4 × π
= 240π cm³
Ratio of Volume of cone (Question 7)/Volume of cone (Question 8)
= 100π/240π
= 5/12
= 5:12

Question 9: A heap of wheat is in the form of a cone whose diameter is 10.5 m and height is 3 m. Find its volume. The heap is to be covered by canvas to protect it from rain. Find the area of the canvas required.
Answer:
Given
Height of the heap = 3 m
Diameter of the heap = 10.5 m
Radius = Diameter/2 = 10.5/2 m
l = √r² + h²
l = √(5.25)²+ (3)²
l = √27.5625 + 9
l = √36.5625
l = 6.04 m (approx)

Volume of the cone = 1/3πr²h
= 1/3 × 22/7 × 10.5/2 × 10.5/2 × 3
= 11 × 1.5 × 10.5/2
= 173.25
= 86.625 m³

Area of the canvas required to cover the heap of wheat = πrl
= 22/7 × 10.5/2 × 6.04
= 11 × 1.5 × 6.04
= 99.66 m²
Therefore, the volume of the heap is 86.625 m³ and the area of the canvas required is 99.66 m².